نمودارهای وننمودارهای ون نمایشهای گرافیکی یا تصویری از روابط بین گروههای مختلف چیزها هستند. نمودار ون توسط جان ون در حدود سال ۱۸۸۰ اختراع شد. نمودارهای ون همچنین به عنوان نمودارهای مجموعه شناخته میشوند. نمودارهای ون نمایشی منطقی از گروهها یا مجموعهها ارائه میدهند. روابط بین دو یا سه مجموعه به راحتی با کمک نمودارهای ون قابل درک است. در ریاضیات، نمودارهای ون برای تحلیل اطلاعات شناخته شدهای که از نظرسنجیها، گزارشهای داده و جداول به دست آمدهاند، استفاده میشوند.
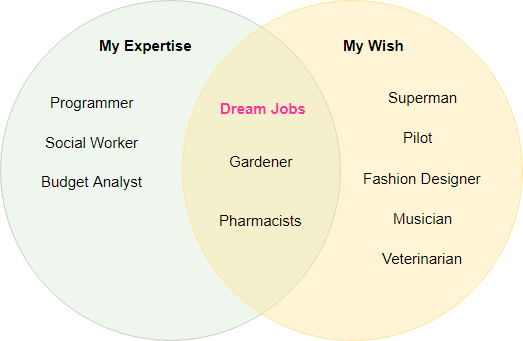
هدف از نمودارهای ون
- نمودارهای ون در نمایش شباهتها یا تفاوتها بین مجموعههای داده متعدد (معمولاً دو تا چهار) مؤثر هستند.
- نمودارهای ون همچنین نتیجه ادغام چندین گروه داده را توصیف میکنند.
- آنها همچنین میتوانند مسئولیتهای همپوشانی دو سازمان/تیم را توصیف کنند.
نمودارهای ون دایرهای
نمودارهای ون از دایرهها برای نمایش مجموعهها و برای نشان دادن رابطه بین یک مجموعه محدود از مجموعههای مختلف استفاده میکنند. در ریاضیات، نمودارهای ون برای تحلیل اطلاعات شناخته شدهای که از نظرسنجیها، گزارشهای داده و جداول که شامل چندین منحنی بسته همپوشان است، استفاده میشوند که معمولاً دایرهها هستند و هر کدام نمایانگر یک مجموعه هستند.
به عنوان مثال:
- مجموعه اعداد طبیعی = {۱،۲،۳،…..}
- مجموعه اعداد صحیح = {۰،۱،۲،۳،…..}
- هر شیء به عنوان یک عنصر از مجموعه نامیده میشود.
مثالهای زیر عملیات پایهای مجموعه را در ارتباط با نمودارهای ون نشان میدهند.
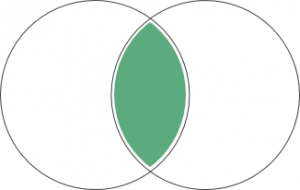
عملیات پایهای مجموعه نمودار ونتقاطع دو مجموعه A ∩B = تعداد عناصری است که در هر دو مجموعه A و B وجود دارند.
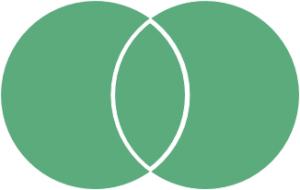
اتحاد دو مجموعه A U B = تعداد عناصری است که در یکی از مجموعههای A یا B وجود دارند.
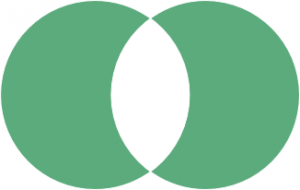
تفاضل متقارن دو
مثالهای نمودار ون
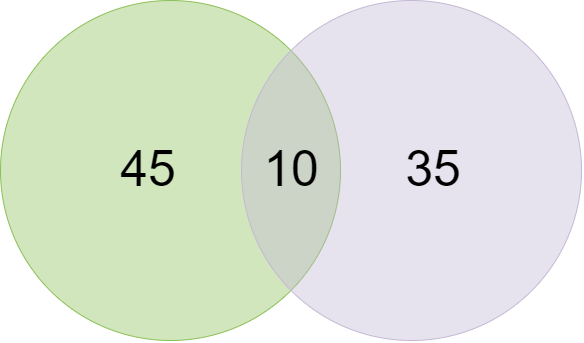
مثال ون: کلاس دانشآموزان
در یک کلاس ۱۰۰ نفری، ۳۵ نفر به علوم علاقه دارند و ۴۵ نفر به ریاضی. ۱۰ نفر به هر دو علاقه دارند. چند نفر به یکی از آنها علاقه دارند و چند نفر به هیچکدام علاقه ندارند؟
- تعداد کل دانشآموزان، n(µ) = ۱۰۰
- تعداد دانشآموزان علوم، n(S) = ۳۵
- تعداد دانشآموزان ریاضی، n(M) = ۴۵
- تعداد دانشآموزانی که به هر دو علاقه دارند، n(M∩S) = ۱۰
- تعداد دانشآموزانی که به یکی از آنها علاقه دارند،
- n(MᴜS) = n(M) + n(S) — n(M∩S)
- → 45+35–10 = 70
- تعداد دانشآموزانی که به هیچکدام علاقه ندارند = n(µ) — n(MᴜS) = ۱۰۰–۷۰ = ۳۰
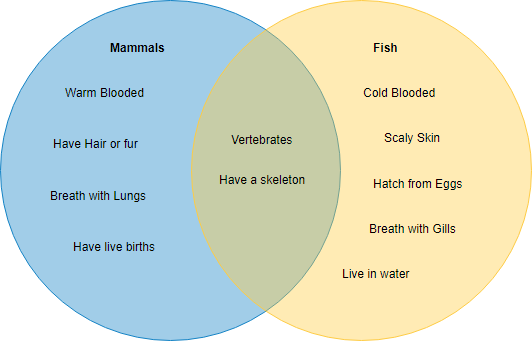
مثال نمودار ون: پستانداران و ماهیها
این یک مثال از نمودار ون است که ویژگیهای پستانداران و ماهیها را نشان میدهد که به عنوان دو بیضی گروهبندی شدهاند. ویژگیهایی که مشترک هستند در منطقه همپوشانی دو بیضی قرار میگیرند.
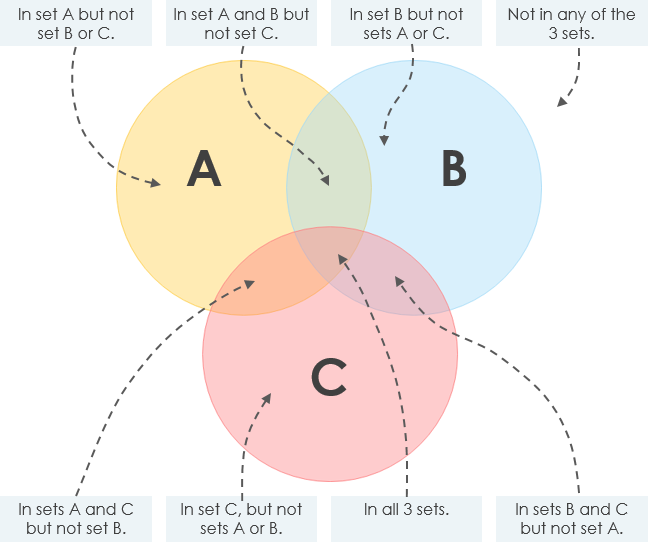
نمودار ون سه دایرهای
یک نمودار ون ۳ دایرهای روشی برای طبقهبندی گروههای اشیاء با ویژگیهای مشابه است.
- این نمودار دارای سه دایره است که با یکدیگر همپوشانی دارند.
- هر دایره مجموعهای از ویژگیهای اشیاء را دارد که به داخل دایره میروند، مانند اعداد فرد یا اشکالی با زوایای راست.
- همچنین فضایی خارج از دایرهها وجود دارد که اشیایی که هیچیک از ویژگیها را ندارند میتوانند در آنجا قرار بگیرند.
- نمودار زیر به شما نشان میدهد که چگونه یک نمودار ون با ۳ دایره کار میکند.
مثال زیر مفهوم مجموعه فوق را نشان میدهد.

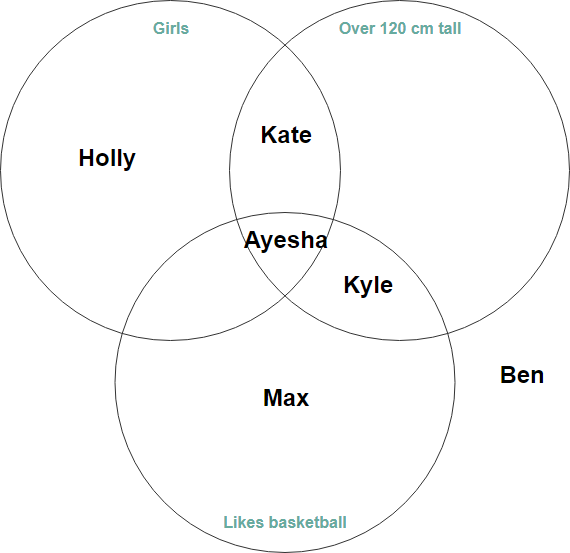
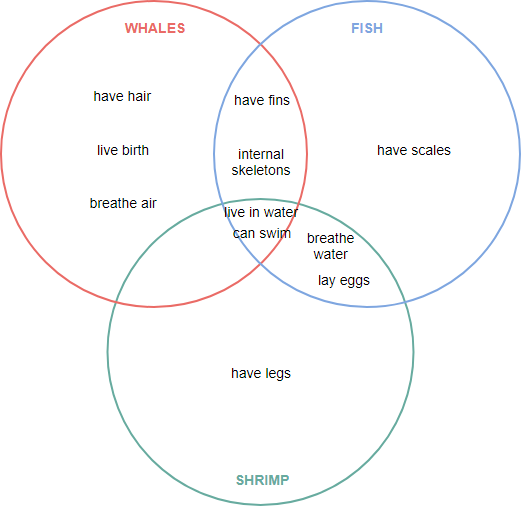
مثال نمودار ون ۳ دایرهای — موجودات آبزی
با گسترش مثال فوق، این نمودار ون برای نشان دادن روابط بین موجودات آبزی: نهنگها (پستانداران) و ماهیها، میگو (موجودات آبزی) استفاده میشود. در زمینههای مختلف ویژگیهای موجودات آبزی وجود دارد. در همپوشانیها، موجودات ویژگیها را به اشتراک میگذارند؛ در خارج از همپوشانیها، موجودات ویژگیهای متفاوتی دارند.
ابزار رایگان آنلاین نمودار ون
آیا میخواهید نمودار ون را به صورت آنلاین با ابزار رایگان ایجاد کنید؟
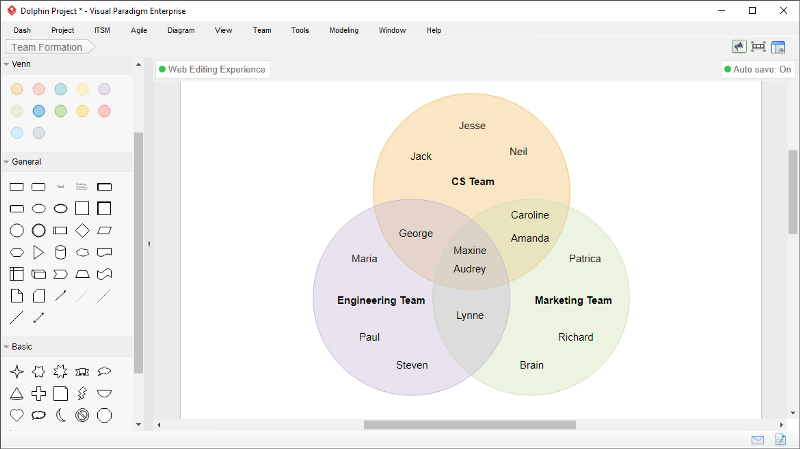
اینجا یکی وجود دارد — ویژوال پارادایم آنلاینیک ابزار ساده برای رسم نمودار است که از طیف وسیعی از نمودارهای فنی و تجاری، از جمله نمودار ون، پشتیبانی میکند.
- نمودار ون را ایجاد کنیدبا کشیدن و رها کردن، اشکال بیضی و متنی را به دقت با راهنمای تراز، تراز و چیدمان کنید و غیره. اینگونه به نظر میرسد
This post is also available in Deutsch, English, Español, Français, Bahasa Indonesia, 日本語, Polski, Portuguese, Ру́сский, Việt Nam, 简体中文 and 繁體中文.













